The Larson Research Center

In the previous posts, we’ve seen how to define the “directions” of natural numbers, by defining number as Hamilton did, as order in progression, instead of increased or diminished magnitude. By taking two of these progression-defined numbers, as the two, reciprocal, aspects of one progression, as Larson did, and by defining two interpretations of these reciprocal numbers, we have been able to establish two groups, one group under addition, analogous to the integers, with an identity element of 0, and one group under multiplication, analogous to the fractions, with an identity element of 1.

In the previous post, we showed how combining the unit magnitudes of the positive and negative “directions” defines a two unit “distance,” or interval, analogous to a spatial distance, with two algebraic “directions,” one negative and one positive:

1|2 + 2|1 = 3|3 = 0
(1|2) = -(2|1)
(2|1) = -(1|2)

However, the fact that the pipe symbol indicates that the reciprocal relation is to be interpreted as the value of the difference (sum of opposite signs) between the numerator and the denominator means that any number n|n can be used as the identity element, and since the quantities in the numerator and denominator are defined in terms of order in progression, rather than as increased, or diminished, quantities, it is necessary to recognize how those quantities differ; that is, how does 1 become 2 and 2 become 3, or 1?

With non-reciprocal numbers defined as magnitudes, 1 becomes 2, when two independent magnitudes are summed:

1 + 1 = 2, 2 + 1 = 3,

which represents an arbitrary action of addition. 

However, with non-reciprocal numbers defined as order in progression, 1 becomes 2 and 2 becomes 3, as the progression proceeds:

1, 2, 3, … 

but what are the dimensions of these steps of progression? Ordinarily, the absence of a superscript with a number indicates that it is 1-dimensional, and we have seen that in ordinary mathematics, any number raised to the zero power is defined by the law of exponents, as the number 1, since all such numbers

n/n = n¹/n¹ = 1^1-1 = 1⁰ = 1.

However, as we saw in the last post, this definition is problematic, theoretically, because it means that the unit cube, 1³, must be defined as

n⁴/n¹ = 1^{4 -1} = 1³ = 1

and since, in a 3D system, we can’t define the four-dimensional unit required to do this, confusion results. Fortunately, we avoid this problem in the mathematics of reciprocal numbers, because the dimensions of the numbers express their inherent dual “directions” (positive and negative), which gives meaning to 1⁰, as a number with no degree of freedom.  So, we simply start with dimension 0, at the top of the tetraktys, meaning there is no, dual, degree of freedom in the initial number of the tetraktys. It simply corresponds to a geometric point. 

Ordinarily, we would regard a progression of reciprocal numbers, with two, reciprocal, aspects, as an ordered series of 0-dimensional units, or scalars, which would constitute a series of points, not 1D lines. Yet, an unexpressed exponent is assumed to equal 1. So, writing the series,

1|1, 2|2, 3|3, … 

implies an exponent of 1 in the numerator and the denominator, but in this case we can’t subtract the exponent of the denominator from the exponent of the numerator, because the pipe symbol indicates that the reciprocal operation of the reciprocal number is not multiplication (division), but addition (subtraction).  Therefore, the exponents must be the same in both cases, because the subtraction operation (actually sum of opposite signs) wouldn’t be valid otherwise, since we can’t subtract (add) two numbers with different exponents, or dimensions. 

Yet, from our knowledge of the tetraktys, we know that the reciprocal of the scalar (dimension 0) is the pseudoscalar (dimension 3, at the 3D level, or bottom of the tetraktys). So, if one of the terms in a reciprocal number is a 0D scalar, the meaning of the pipe symbol, “|”, requires the other term to be the reciprocal of the scalar, the pseudoscalar! 

We can see that this makes sense, because the series of reciprocal numbers

1⁰|1⁰, 2⁰|2⁰, 3⁰|3⁰, …  =  0⁰, 0⁰, 0⁰, …

is meaningless. A point is only its own reciprocal, when no degree of freedom is present (the n⁰:n⁰ numbers at the top of the tetraktys). Its reciprocal, with any non-zero degree of freedom, is always the pseudoscalar. Hence, the 3D pseudoscalar is the appropriate reciprocal of the 0D scalar in the Euclidean space (i.e. the 2³ numbers at the bottom of the tetraktys).

Consequently, in the three-dimensional system of numbers (the Grassmann algebra), the progression of reciprocal numbers must take the form 

1³|1⁰, 2³|2⁰, 3³|3⁰, …

which is a series of reciprocal numbers expressing a numerical progression of cubes, combined with reciprocally related points, corresponding to the geometric structure of Larson’s cube, with the 0D scalar at the intersection of the stack of 2x2x2 cubes.

However, because the difference between the numerator and the denominator is the difference between reciprocal quantities of different dimensions, we can express its value, as some mathematically meaningful result, only if the denominator is always the 0D scalar, while the numerator is the, reciprocal, pseudoscalar, since subtracting 0 from anything is essentially meaningless, and the breaking of the rule of exponents has no consequences in this case. As they say in the gym, no harm, no foul. 

However, if we change from the pipe operation to the slash operation, then, according to the same mathematical rules, it’s possible to express the operational result as a meaningful quantity. That is to say,

1³/1⁰, 2³/2⁰, 3³/3⁰, … = 1^3-0, 1^3-0, 1^3-0, … = 1³, 1³, 1³, …

Why is this? I submit that it’s because, in the slash operation, the ratio of reciprocals, as a quotient, defines the unit of a function. So, 1³ is a cubic unit of the function, which equates to a cubic pseudoscalar unit per scalar unit. On the other hand, in the pipe operation, 1³|1⁰, the ratio of reciprocals defines the unit of volume, as a 3D interval, with eight directions, between the 0D point and the 3D cube. 

This difference between the two operations enables us to distinguish, in an important manner, the difference between scalar magnitudes of motion, with two “directions,” and vector magnitudes of motion, in two directions. The difference in the magnitudes is the difference in the point of reference. We represent the opposite direction of a vector, by placing the arrow head at the opposite end of the line:

—————————> or <——————————

However, we represent the opposite “directions” of scalars, by placing the arrow head at both ends of a line, pointing in opposite “directions,” like this:

<————————>

This is because motion, as a 1D scalar magnitude, is an expansion from the center outward, in opposite directions, while motion, as a 1D vector magnitude, is a transference from one end of a line to the other. Thus, a scalar line always has a middle point associated with it, which is not part of a vector line. Therefore, the reciprocal number,

1¹|1⁰ = 1 

is a numerical expression of the double headed arrow

<————-0————->

or the result, or interval, we might say, of a 1D scalar expansion outward from a point.

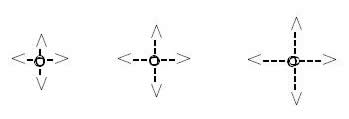
By the same token, the reciprocal number,

1²|1⁰ = 1²

is a numerical expression of the four headed arrow

or the result of a 2D expansion from a point.

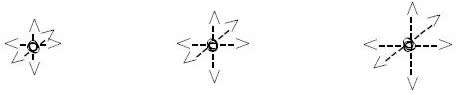
Finally, the reciprocal number

1³|1⁰ = 1³

is a numerical expression of the six headed arrow

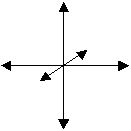
or the result of a 3D expansion from a point.

The important difference in scalar motion versus vector motion is that the two “directions” in one dimension of scalar motion produce two 1D scalar magnitudes (one in each “direction”), in one unit of time, the four “directions” in two dimensions of scalar motion produce four 2D scalar magnitudes, in one unit of time, while the six “directions” in three dimensions of scalar motion produce eight 3D scalar magnitudes, in one unit of time.

This means that to represent the unit progression of the RST, with reciprocal numbers, we write the series

1³:1⁰, 2³:2⁰, 3³:3⁰, …

where the colon symbol for ratio is used as a general symbol for reciprocity, which can be interpreted as either of the two operations we have defined. Consequently, this gives us two representations of the reciprocal operation: One is a geometric interval, and the other is a function, which produces that interval; that is, one is a representation of a scalar “distance” with two fixed, reciprocal, aspects, the scalar and pseudoscalar, while the other is a representation of a function, with two changing, reciprocal, aspects, the scalar and pseudoscalar.

On this basis, the 0D scalar progression, or scalar expansion of a point, is

1⁰:1⁰, 2⁰:2⁰, 3⁰:3⁰, … = 1⁰, 2⁰, 3⁰, …

where the expanded scalar intervals, i_n, are

i_n = 1⁰|1⁰, 2⁰|2⁰, 3⁰|3⁰, … = 1⁰, 2⁰, 3⁰… (0, 0, 0,…)

And the function of the scalar progression, f(p⁰), which produces them, is

f(p⁰) = Δ1⁰/Δ1⁰.

The 1D scalar progession, or scalar expansion, of a line, is 

1¹:1⁰, 2¹:2⁰, 3¹:3⁰, … = 1¹, 2¹, 3¹, …

where the expanded scalar intervals are

i_n = 1¹|1⁰, 2¹|2⁰, 3¹|3⁰, … = 1¹, 2¹, 3¹ (<-0->, <—0—>, <—-0—->, …)

And the scalar function, which produces them, is

f(p¹) = Δ1¹/Δ1⁰

However, notice that this time, due to the fact that there are TWO directions in the ONE dimension, the progression of the 1D units, as opposed to the progression of the 0D units, is an increase in multiples of two 1D units, one “positive” unit, relative to zero, and one negative unit, relative to zero: 2, 4, 6, …, or the 1D progression, P¹, is P¹ = (2*1¹), (2*2¹), (2*3¹), …

Now, the 2D scalar progession, or scalar expansion, of an area, is 

1²:1⁰, 2²:2⁰, 3²:3⁰, … = 1², 2², 3², … 

where the expanded scalar intervals are

i_n = 1²|1⁰, 2²|2⁰, 3²|3⁰, … = 1², 2², 3², …

And the scalar function, which produces them, is

f(p²) = Δ1²/Δ1⁰

Again, however, due to the fact that there are TWO directions in each of the TWO dimensions, the progression of the 2D units, as opposed to the progression of the 1D units, is an increase in multiples of four 2D units, two polarized units in two independent directions, relative to zero, and two oppositely polarized units in two opposite independent directions, relative to zero: 4, 16, 36, …, or the 2D progression, P², is P²  = (4*1²), (4*2²), (4*3²), …

Finally, the 3D scalar progession, or scalar expansion, of a volume, is 

1³:1⁰, 2³:2⁰, 3³:3⁰, … = 1³, 2³, 3³, …    

where the expanded scalar intervals are

i_n = 1³|1⁰, 2³|2⁰, 3³|3⁰, … = 1³, 2³, 3³, …  

And the scalar function, which produces them, is

f(p³) = Δ1³/Δ1⁰

Now, due to the fact that there are TWO directions in each of the THREE dimensions, the progression of the 3D units, as opposed to the progression of the 2D units, is an increase in multiples of eight 3D units, four polarized units in three independent “positive” directions, relative to zero, and four polarized units in three independent “negative” directions, relative to zero: 8, 64, 216, …, or the 3D progression, P³, is P³ = (8*1³), (8*2³), (8*3³), …

Of course, in the context of the RST, this immediately raises the possibility of the inverse of these intervals, and the functions, which produce them; that is, it is the progression of the temporal tetraktys, in the form of the temporal 2x2x2 stack of cubes. Would this take the form of

f(p^-n) = Δ1^-n/Δ1⁰? 

This is heavy stuff!