The Structure of the Physical Universe
Material Sector Combinations
Combinations of Motion
Description:
In the theoretical universe of nothing but motion, all theoretical entities are either motions, combinations of motions, or relations between motions. However, there are different types of motion. There is the intrinsic motion of matter that constitutes the structure of material entities located at different locations of space and time, possessing the properties of scalar motion, such as mass, charge, spin, etc, and then there is the external motion of matter that constitutes a change of the space and time locations of material entities, possessing the properties of vectorial motion, such as acceleration, momentum and kinetic energy. In addition, there is a third type of motion, the combination of the intrinsic/external scalar motion of matter that constitutes both the structure of material entities, located at different locations of space and time, and a change of their space and time locations. These entities possess the properties of this motion, frequency, momentum, and radiation energy.
Each of these types of motions in the material sector of the universe has its corresponding type in the cosmic sector, where the dimensions of space/time are the dimensions of time/space. However, since energy plays such a central role in physics, and it has the dimensions of time/space, the space and time dimensions in the definitions of LST physics concepts such as energy, momentum, and mass are confusing, when applied to RST physics concepts, without clarification, because, in RST physics, the additional concepts of cosmic energy, momentum, and mass have the same dimensions as their material counterparts. Hence, the following RST definitions are used at the LRC (d=delta):
- Energy (E) = dt/ds
- Momentum (p) = dt2/ds2
- Mass (m) = dt3/ds3
- Velocity (v) = ds/dt
- Inverse momentum (pbar) = ds2/dt2
- Inverse mass (mbar) = ds3/dt3
However, the symbol for delta, ‘d’, in the above definitions has a different meaning, depending upon the type of motion with which these n-dimensional forms of motion are associated. In vectorial motion, for instance, the space delta is “taken to the limit” in the definition of velocity, because any value of vectorial motion is possible, ranging from zero change in location, to just under c-speed change in location.
This means that the value of the ds/dt ratio may take any value during a given interval of time, and, so, to minimize the calculation error that would be introduced, if the space/time ratio does vary in a given time interval, the space and time interval are minimized (taken to the limit) in the definition of vectoral motion, for the purpose of carrying out calculations, using Newton’s and Liebniz’s differential calculus. Thus, velocity, ds/dt, in the context of vectorial motion, is regarded as the “derivative of s with respect to t,” and the complicated processes of finding it is called finding a derivative, or differentiating. The ds’s and dt’s, appearing separately, are called differentials.
Clearly, this is not the case in scalar motion, where velocity is defined as the ratio of the space/time aspects in a progression, or a constant increase (decrease) of space and time, which never varies. Thus, ds/dt, in the definition of scalar motion velocity, cannot be taken to the limit approaching zero, because zero scalar motion doesn’t exist. The minimum increments of space and time are set by the value of the natural unit of motion, and the value selected as the natural unit of space, or time. In our case, we have selected c-speed as the natural unit of motion, and a natural unit of time based on the Rydberg constant of hydrogen, as explained in the
magnitudes section of the SPUD. Hence, the unit of natural motion magnitude is absolute in the context of scalar motion, and ds/dt has a fixed, unit, value.
There are two unit values possible, as explained in the
Formation section of the SPUD. These are the space unit-displacement ratio (SUDR), ds|dt = 1|2, and the time unit-displacement ratio (TUDR), ds|dt = 2|1. Multiples of these units, n|2n and 2n|n, may combine to form SUDR|TUDR combinations, or S|T units. We can express this operation algebraically as
S|T = x|2x + 2y|y.
However, due to the physical nature of the “direction” reversals, which create the space and time displacements in the SUDRs and TUDRs, the above equation is incomplete in that it only contains the value of the increasing portion of the reversing aspect’s cycle of progression. To obtain the correct value, we need to also include the value of the decreasing portion of the cycle of both unit displacement ratios (UDRs). On this basis, we obtain
S|T = x|2x + x|y + 2y|y = 2x+2y|2x+2y
Consequently, the minimum unit is formed when x = y = 1,
S|T = 1|2 + 1|1 + 2|1 = 4|4.
Because S|T units progress in both space and time, they can encounter other UDRs, both SUDRs and TUDRs that progress either in time, or space, only, which means the values of x and y are determined by probability principles. This is illustrated in the world line chart shown in figure 1 below.
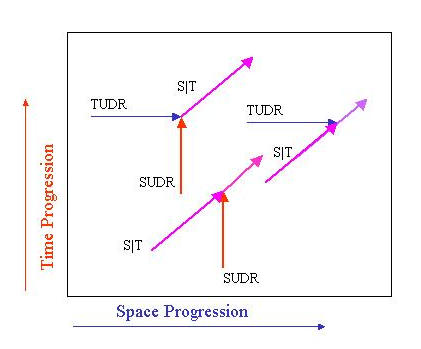
Figure 1. Three Interactions of SUDRs, TUDRs, and SUDR|TUDR (S|T) Units
As indicated by the colors of the entities in the figure above, when a red SUDR (indicating “unidirectional” time progression) encounters a blue TUDR (indicating “unidirectional” space progression) in the temporal future of its space location (or vice versa), the resulting combination progresses in both space and time, as a c-speed S|T unit. The color magenta indicates the operation of two 1D entities combining into one 2D entity; that is, the magenta color is a result of combining one “red” and one “blue” unit:
S|T = 1|2 + 1|1 + 2|1 = 4|4
On the other hand, when an existing S|T unit encounters either a SUDR or TUDR in its spatial, or temporal, progression, its number of SUDRs (x|2x), or TUDRs (y|2y), is increased, as indicated by the change of S|T color in the red, or blue, “direction.”
Clearly, the middle term, the value of the inward component of the x number of SUDRs and the y number of TUDRs, is a 2D value, since x and y are independent values. The value of x in this term is inward space progression and can vary from 1 to infinity, depending upon the number of SUDRs, while the value of y is inward time progression and can also vary from 1 to infinity, depending upon the number of TUDRs, in the S|T unit.
Hence, while the SUDRs and TUDRs are discrete, one-dimensional units (single term entities), with both an outward and inward component, the S|T combo unit is a 2D entity, with both an outward and inward component to its progression. This conservation of the scalar “direction” duality, as the dimension of the combinations increases, corresponds to the conservation of vectorial direction duality, as the number of vector dimensions increase.
In the case of vectors, we don’t explicitly recognize the duality of points (except in non-Euclidean geometry), but in the 3D vector tetraktys, and in its corresponding 3D algebra, geometric algebra (GA), the sphere and point are clearly dual, as implied by the terms scalar and pseudoscalar. The dual of each of the three basis vectors is its reflection, called orientation in GA. The dual of each of the three basis bivectors is the opposite, or reflected, side of the plane, indicated as an orientation of rotational direction. Interestingly, the dual of the trivector is indicated in GA by an outward or inward direction (orientation) of the sphere, relative to its origin, or point.
Similarly, in the 3D scalar tetraktys of colors, the duality of the black “point” is implied by the white “sphere,” but what is the duality of the 1D red, blue and green colors and the 2D magenta, cyan, and yellow colors? The fact is, there is no way, using colors, as a scalar analogy of the vector tetraktys, to indicate the duality of colors, unless we treat color as the electromagnetic frequency that it is, then choose a “unit” frequency, and modulate it, and in this way produce the positive and negative frequencies of the modulated carrier, each with their two side bands.
However, to do this would be much more involved than it’s worth, at this point, requiring the mathematics of Fourier transforms, etc. Instead, we will use the “directional” values of red and blue to indicate the negative and positive values of SUDRs and TUDRs, respectively. Thus, the dual of the 1D red is blue, and the dual of outward magenta is inward magenta, which as we have just seen above, corresponds to the scalar “directions” of the mathematical terms in the SUDRs, TUDRs, and S|T units. Consequently, when S|T units are combined, each unit’s dual magenta value, both outward and inward, will be conserved.
