The Larson Research Center

Assuming a 3D oscillation of space and an inverse 3D oscillation of time, called space unit displacement ratio (SUDR) and time unit displacement ratio (TUDR), respectively, we have combined them into SUDR|TUDR (S|T) units, which we then use as preons to construct a model of particles corresponding to the particles of the LST community’s standard model of particle physics.

Of course, the standard model is based on the Newtonian system of theory, which, based on vector motion, constitutes his program of research to find the fewest number of particle interactions among the fewest number of particles, in the structure of the physical universe.

Though the LST community has successfully modified the concept of vector motion considerably, substituting the description of a particle’s state, by means of its “probability amplitude,” in space and time, for the description of a particle’s state, by means of its change in position, in space and time, they have done so with an algebra Sir William Rowan Hamilton regarded as non-intuitive, unscientific and even self-contradictory, in contrast to Euclidean geometry (see here.)

Larson excluded the RST community from using this defective algebra, when he formulated the second fundamental postulate and included in it the assumptions of Euclidean geomety and the “relations of ordinary commutative mathematics.”

Some have argued that the word “ordinary” is too vague a term, that he should have been more explicit, but it’s clear that he was referring to the unfamiliar rule for multiplication that had emerged in connection with quantum physics, during his formative years. It was this rule, the rule of non-commutative multiplication, where p times q is not equal to q times p, which was known to mathematicians familiar with matrix multiplication, but not to most physicists, which enabled the science of quantum  mechanics from that point on.

It was based on multiplying the amplitudes of two oscillations in order to calculate the energy involved. If the rule of ordinary commutative mathematics were used in these calculations, the result was an infinite sum in a Fourier series. The solution was to use non-commutative multiplication, something that disconcerted physicists, but not mathematicians.

Larson was good at mathematics, but he was not interested in the philosophy of the subject. He was interested in getting away from the necessity of using partial differential equations (PDEs), and the attendant “playing with variables” to find solutions to physical questions. He sincerely believed that there had to be a better way and he sought to find it in the observable world of Euclidean geometry, absolute magnitudes and ordinary commutative mathematics. 

However, as we have studied the consequences of the RST postulates at the LRC, and in working to develop a new theory based on them, we have discovered the work of Hamilton in mathematics, the very man who coined the terms “vector” and “quaternion.” In the process, we discovered the work of Hestenes, who laid out the development of algebra, from the days of Hamilton, in terms of key concepts of Grassmann and Clifford, which has led to our ideas of unit time and space displacements, which have two interpretations, the quantitative (-1, 0, +1), and operational (1/2, 1/1, 2/1) interpretations.

All of this was studied in terms of the tetraktys, which ended up allowing us to unify the mathematical concepts of magnitude, dimension and “direction,” with the corresponding geometrical concepts of point, line, area and volume. The effort ever since then has been to understand our RST-based physical theory in terms of this unified view of mathematics and geometry.

The result has been gratifying to say the least. We have even received a couple of substantial private awards, from those who have followed our efforts and wanted to encourage the work, but, now, we are at the point of having to understand how to add, subtract, multiply and divide with the numbers of the tetraktys that we have developed. We need an “ordinary commutative mathematics,” a multi-dimensional, scalar, algebra, if you will, to enable us to combine units of scalar motion and calculate the relations between them. In short, we need an algebra of multi-dimensional scalar motion.

With an algebra of multi-dimensional scalar motion, we can combine our preons into the bosons of photons, and then combine these into fermions of quarks and leptons, and then combine these into the elements of matter and anti-matter. Such an algebra will enable us to calculate the properties of each of these entities with a precision equal to, if not greater than, the precision obtained in quantum mechanics, but with unprecedented conceptual clarity.

We have made a lot of progress in establishing the fundamentals of the scalar algebra. One important milestone is the identification of the multi-dimensional numbers of the tetraktys with the geometric structure of Larson’s Cube (LC), which has enabled us to describe the S|T units in terms of discrete and continuous magnitudes. This way, we can do calculations, using the discrete numbers of the LC’s lines, squares and cubes, and then we can transform these into the corresponding continuous numbers that we have found in the ratios of its nested radii, surfaces and volumes.

An important indication that we are not wasting our time is the recent derivation of Einstein’s famous mass —> energy equation from the fundamental components of the S|T units. This was done by simply writing the two reciprocal units of the S|T unit as follows:

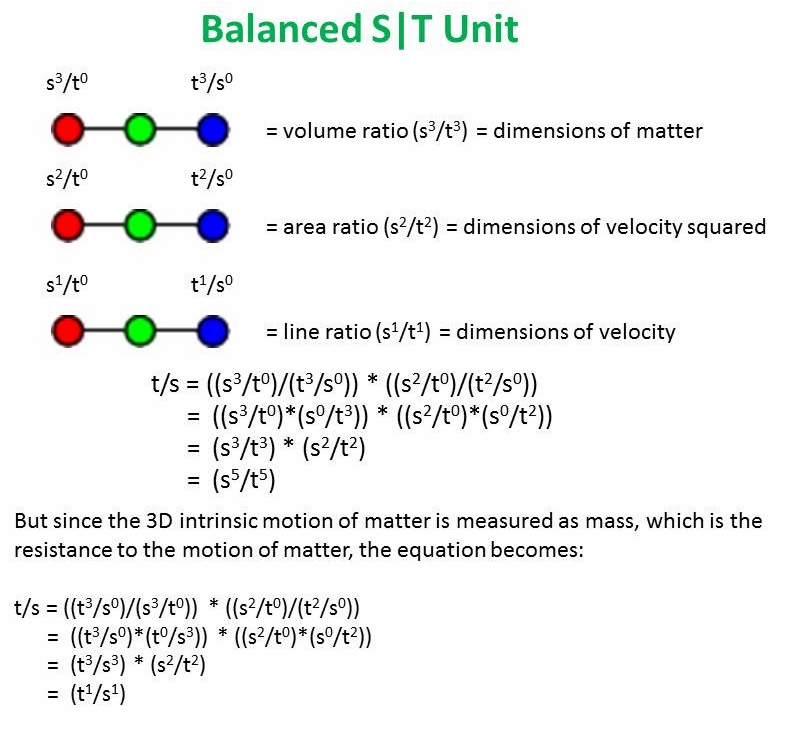
Figure 1. Einstein’s Equation in Terms of the S|T Unit of Scalar Motion

Since the photon in the LRC’s theory consists of one or more of these S|T units, we now have to calculate the different frequencies possible and their associated energy and how these bosons relate to the fermions, but it’s interesting to see that the properties of the preon of the fermion, photons in our theory, conform to the fundamental energy equation of mass and energy, as shown here.